Lecture 03: 3D to 2D projections

Computer graphics in Game development

Ivan Belyavtsev

23.10.2020

What is a rasteriztion

It’s possible to construct any image using the next operation:

draw a point
draw a line**
draw a triangle

Rendering pipeline

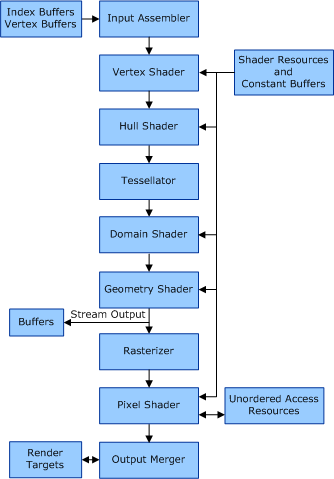
From: https://docs.microsoft.com/en-us/windows-hardware/drivers/display/pipelines-for-direct3d-version-11

3D to 2D conversation pipeline

From model space to world space
From world space to view space (camera space)
From view space to projection space
From projection space to clipping space
From clipping space to homogeneous screen space
From homogeneous screen space to screen space [1]

Spaces

Model space - coordinate system where the object was created
World space - where all object located in proper locations
View space - a space located around camera
Projection space - a projected camera frustrum to cube space
Screen space - a space of resulted image [2]

Homogeneous coordinates

Homogeneous coordinates - cartesian coordinates + 1 dimension

\[ (X, Y, Z) => (x, y, z, w) \]

\[ X = x/w, Y = y/w, Z = z/w \] [3]

Transformations in homogeneous coordinates

\[T(\overrightarrow{x}) = A\overrightarrow{x}\]

where \[A = \left[\begin{array}{cccc} x1 & x2 & x3 & x4 \\ y1 & y2 & y3 & y4 \\ z1 & z2 & z3 & z4 \\ w1 & w2 & w3 & w4 \end{array}\right]\] [4]

Translation in homogeneous coordinates

\(\overrightarrow{tr}\) - vector of translation

\[T = \left[\begin{array}{cccc} 1 & 0 & 0 & tr.x \\ 0 & 1 & 0 & tr.y \\ 0 & 0 & 1 & tr.z \\ 0 & 0 & 0 & 1 \end{array}\right]\] [4]

Scaling in homogeneous coordinates

\(\overrightarrow{scale}\) - vector of scaling

\[S = \left[\begin{array}{cccc} scale.x & 0 & 0 & 0 \\ 0 & scale.y & 0 & 0 \\ 0 & 0 & scale.z & 0 \\ 0 & 0 & 0 & 1 \end{array}\right]\] [4]

Rotation around X

\(\alpha\) - angle of rotation around axis X

\[R_x = \left[\begin{array}{cccc} 1 & 0 & 0 & 0 \\ 0 & cos(\alpha) & -sin(\alpha) & 0 \\ 0 & sin(\alpha) & cos(\alpha) & 0 \\ 0 & 0 & 0 & 1 \end{array}\right]\] [4]

Rotation around Y

\(\beta\) - angle of rotation around axis Y

\[R_y = \left[\begin{array}{cccc} cos(\beta) & 0 & sin(\beta) & 0 \\ 0 & 1 & 0 & 0 \\ -sin(\beta) & 0 & cos(\beta) & 0 \\ 0 & 0 & 0 & 1 \end{array}\right]\] [4]

Rotation around Z

\(\gamma\) - angle of rotation around axis Z

\[R_z = \left[\begin{array}{cccc} cos(\gamma) & -sin(\gamma) & 0 & 0 \\ sin(\gamma) & cos(\gamma) & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 1 \end{array}\right]\] [4]

World transformations

\[World=TRS\]

First scale, then rotate, then translate

Camera model

\(\overrightarrow{Eye}\) - camera location vector
\(\overrightarrow{At}\) - camera look-at target
\(\overrightarrow{Up}\) - camera up vector (or world up direction)

View transformations

\[ \overrightarrow{zaxis} = \frac{\overrightarrow{Eye} - \overrightarrow{At}}{||\overrightarrow{Eye} - \overrightarrow{At}||} \] \[ \overrightarrow{xaxis} = \frac{\overrightarrow{Up} \times \overrightarrow{zaxis}}{||\overrightarrow{Up} \times \overrightarrow{zaxis}||}\] \[ \overrightarrow{yaxis} = \overrightarrow{zaxis} \times \overrightarrow{xaxis} \]

View transformations

\[View = \left[\begin{array}{cccc} xaxis.x & yaxis.x & zaxis.x & 0 \\ xaxis.y & yaxis.y & zaxis.y & 0 \\ xaxis.z & yaxis.z & zaxis.z & 0 \\ \overrightarrow{xaxis} \cdot \overrightarrow{eye} & \overrightarrow{yaxis} \cdot \overrightarrow{eye} & \overrightarrow{zaxis} \cdot \overrightarrow{eye} & 1 \end{array}\right]\]

Projection parameters

\(S_w\) - screen window width in camera space in near clipping plane
\(S_h\) - screen window height in camera space in near clipping plane
\(Z_n\) - distance to the near clipping plane along Z axes in camera space
\(Z_f\) - distance to the far clipping plane along Z axes in camera space

Orthogonal projection

\[Projortho = \left[\begin{array}{cccc} \frac{2}{S_w} & 0 & 0 & 0 \\ 0 & \frac{2}{S_h} & 0 & 0 \\ 0 & 0 & \frac{1}{Z_f-Z_n} & 0 \\ 0 & 0 & \frac{-Z_n}{Z_f-Z_n} & 1 \end{array}\right]\]

Perspective projection

\[Proj = \left[\begin{array}{cccc} \frac{2Z_n}{S_w} & 0 & 0 & 0 \\ 0 & \frac{2Z_n}{S_h} & 0 & 0 \\ 0 & 0 & \frac{Z_f}{Z_f-Z_n} & 1 \\ 0 & 0 & \frac{-Z_n\cdot Z_f}{Z_f-Z_n} & 0 \end{array}\right]\]

Viewport

\[Viewport = \left[\begin{array}{cccc} width & 0 & 0 & 0 \\ 0 & -height & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 1 \end{array}\right]\]

Chain transitions together

\[\vec{X} = Viewport \cdot Proj \cdot View \cdot World \cdot \vec{x}\]

Dangerous zone

Remember about left-handed and right-handed operations!

Check correctness of each matrix, e.x. in DirecX documentation [1]

“Projection” experiment

Deduction phase

All verteces need proper transformation
What about performance?

“Projection” experiment

Experiment

Let’s implement it together

“Projection” experiment

Reference

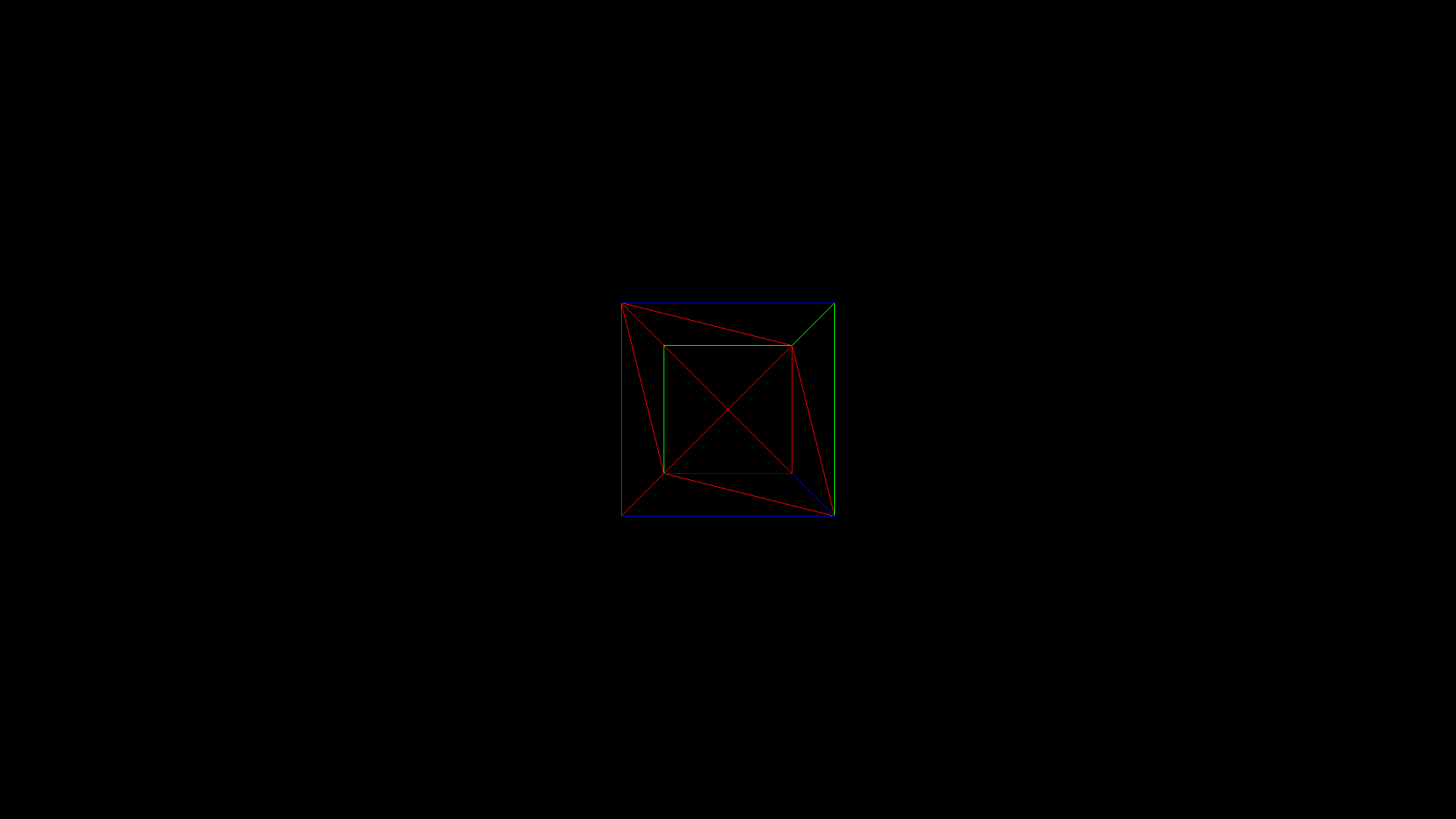
From: https://github.com/djbelyak/basic-of-rasterization/blob/main/references/projection.png

“Projection” experiment

What is the new knowledge?

Proper way to map 3D verteces into image

References

Satran M., Jacobs M. The Direct3D transformation pipeline [Electronic resource]. 2019. URL: https://docs.microsoft.com/en-us/windows/win32/dxtecharts/the-direct3d-transformation-pipeline.

Serrano H. From model space to screen space- OpenGL space transformations [Electronic resource]. 2015. URL: https://www.haroldserrano.com/blog/from-model-space-to-screen-space-opengl-space-transformations.

Jia Y.-B. Homogeneous coordinates // Handout of the Problem Solving Techniques for Applied Computer Science Lecture at Iowa State University. 2014. Vol. 58.

Alamia M. World, view and projection transformation matrices [Electronic resource]. URL: http://www.codinglabs.net/article_world_view_projection_matrix.aspx.